AP Board Class 10 Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions PDF: Download Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Book Answers

AP Board Class 10 Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions PDF: Download Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Book Answers

AP Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks Solutions and answers for students are now available in pdf format. Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Book answers and solutions are one of the most important study materials for any student. The Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.2 books are published by the Andhra Pradesh Board Publishers. These Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 textbooks are prepared by a group of expert faculty members. Students can download these AP Board STD 10th Maths Chapter 6 Progressions Ex 6.2 book solutions pdf online from this page.

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks Solutions PDF

Andhra Pradesh State Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Books Solutions with Answers are prepared and published by the Andhra Pradesh Board Publishers. It is an autonomous organization to advise and assist qualitative improvements in school education. If you are in search of AP Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Books Answers Solutions, then you are in the right place. Here is a complete hub of Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.2 solutions that are available here for free PDF downloads to help students for their adequate preparation. You can find all the subjects of Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks. These Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks Solutions English PDF will be helpful for effective education, and a maximum number of questions in exams are chosen from Andhra Pradesh Board.

Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Books Solutions

Board	AP Board
Materials	Textbook Solutions/Guide
Format	DOC/PDF
Class	10th
Subject	Maths
Chapters	Maths Chapter 6 Progressions Ex 6.2
Provider	Hsslive

How to download Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions Answers PDF Online?

1. Visit our website - Hsslive
2. Click on the Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Answers.
3. Look for your Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks PDF.
4. Now download or read the Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions for PDF Free.

AP Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks Solutions with Answer PDF Download

Find below the list of all AP Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions for PDF’s for you to download and prepare for the upcoming exams:

10th Class Maths 6th Lesson Progressions Ex 6.2 Textbook Questions and Answers

Question 1.
Fill in the blanks in the following table, given that ‘a’ is the first term, d the common difference and an the nth term of the A.P: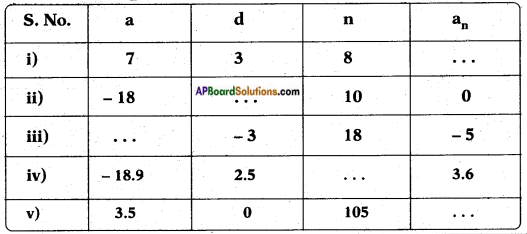

Answer: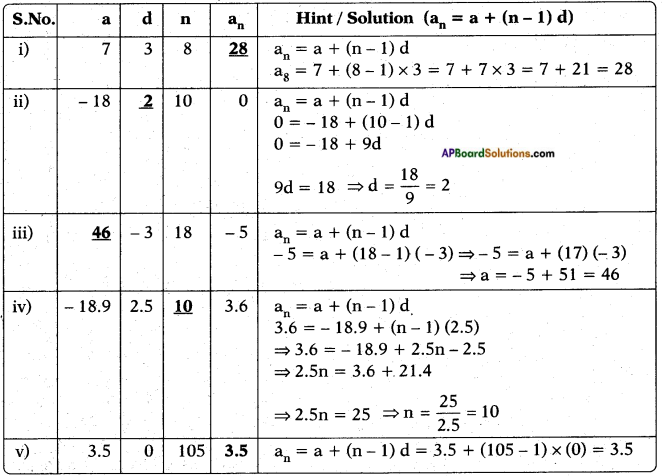

Question 2.
Find the i) 30th term of the A.P.: 10, 7, 4,……
ii) 11th term of the A.P.: -3, –12, 2,…
Answer:
i) Given A.P. = 10, 7, 4, …….
a₁ = 10; d = a₂ – a₁ = 7 – 10 = – 3
a_n = a + (n – 1) d
a₃₀ = 10 + (30 – 1) (- 3) = 10 + 29 × (- 3) = 10 – 87 = – 77

ii) Given A.P. = – 3, –12, 2,…
a₁ = -3; d = a₂ – a₁ = –12 – (-3) = – 3
= –12 + 3
= −1+62
= 52
a_n = a + (n – 1) d
= -3 + (11-1) × 52
= -3 + 10 × 52
= -3 + 5 × 5
= -3 + 25
= 22

Question 3.
Find the respective terms for the following APs.
i) a₁ = 2; a₃ = 26, find a₂.
Answer:
Given: a₁ = a = 2 …….. (1)
a₃ = a + 2d = 26 …….. (2
Equation (2) – equation (1)
⇒ (a + 2d) – a = 26 – 2
⇒ 2d = 24
d = 242 = 12
Now a₂ = a + d = 2 + 12 = 14

ii) a₂ = 13; a₄ = 3, find a₁, a₃.
Answer:
Given: a₂ = a + d = 13 ….. (1)
a₄ = a + 3d = 3 ….. (2)
Solving equations (1) and (2);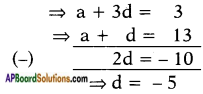

∴ Substituting d = – 5 in equation (1) we get
a + (-5) = 13
∴ a = 13 + 5 = 18 i.e., a₁ = 18
a₃ = a + 2d = 18 + 2(- 5)
= 18 – 10 = 8

iii) a₁ = 5; a₄ = 912, find a₂, a₃.
Answer:
Given: a₁ = a = 5 ….. (1)
a₄ = a + 3d = 912 ….. (2)
Solving equations (1) and (2);

⇒ 3d = 412
⇒ 3d = 92
⇒ d = 92×3 = 32
∴ a₂ = a + d = 5 + 32 = 132
a₃ = a + 2d = 5 + 2 × 32 = 5 + 3 = 8

iv) a₁ = -4; a₆ = 6, find a₂, a₃, a₄, a₅.
Answer:
Given: a₁ = a = -4 ….. (1)
a₆ = a + 5d = 6 ….. (2)
Solving equations (1) and (2);
(-4) + 5d = 6
⇒ 5d = 6 + 4
⇒ 5d = 10
⇒ d = 105
Now
∴ a₂ = a + d = -4 + 2 = -2
a₃ = a + 2d = -4 + 2 × 2 = -4 + 4 = 0
a₄ = a + 3d = -4 + 3 × 2 = -4 + 6 = 2
a₅ = a + 4d = -4 + 4 × 2 = -4 + 8 = 4

v) a₂ = 38; a₆ = -22, find a₁, a₃, a₄, a₅.
Answer:
Given: a₂ = a + d = 38 ….. (1)
a₆ = a + 5d = -22 ….. (2)
Subtracting (2) from (1) we get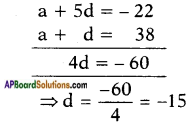

Now substituting, d = – 15 in equation (1), we get
a + (- 15) = 38 ⇒ a = 38 + 15 = 53
Thus,
a₁ = a = 53;
a₃ = a + 2d = 53 + 2 × (- 15) = 53 – 30 = 23;
a₄ = a + 3d = 53 + 3 × (- 15) = 53 – 45 = 8;
a₅ = a + 4d = 53 + 4 × (- 15) = 53 – 60 = – 7

Question 4.
Which term of the AP:
3, 8, 13, 18,…, is 78?
Answer:
Given: 3, 8, 13, 18, ……
Here a = 3; d = a₂ – a₁ = 8 – 3 = 5
Let ‘78’ be the nth term of the given A.P.
∴ a_n = a + (n – 1) d
⇒ 78 = 3 + (n – 1) 5
⇒ 78 = 3 + 5n – 5
⇒ 5n = 78 + 2
⇒ n = 802 = 16
∴ 78 is the 16th term of the given A.P.

Question 5.
Find the number of terms in each of the following APs:
i) 7, 13, 19, ….., 205
Answer:
Given: A.P: 7, 13, 19, ……….
Here a₁ = a = 7; d = a₂ – a₁ = 13 – 7 = 6
Let 205 be the n^th term of the given A.P.
Then, a_n = a + (n – 1) d
205 = 7 + (n- 1)6
⇒ 205 = 7 + 6n – 6
⇒ 205 = 6n + 1
⇒ 6n = 205 – 1 = 204
∴ n = 2046 = 34
∴ 34 terms are there.

ii) 18, 1512, 13, …, -47
Answer:
Given: A.P: 18, 1512, 13, …….
Here a₁ = a = 18;
d = a₂ – a₁ = 1512 – 18 = -212 = –52
Let ‘-47’ be the nth term of the given A.P.
a_n = a + (n – 1) d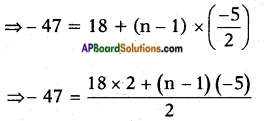

⇒ -94 = 36 – 5n + 5
⇒ 5n = 94 + 41
⇒ n = 1355 = 27
∴ 27 terms are there.

Question 6.
Check whether, -150 is a term of the AP: 11, 8, 5, 2…
Answer:
Given: A.P. = 11, 8, 5, 2…
Here a₁ = a = 11;
d = a₂ – a₁ = 8 – 11 = -3
If possible, take – 150 as the n^th term of the given A.P.
a_n = a + (n – 1) d
⇒ -150 = 11 + (n – 1) × (-3)
⇒ -150 = 11 – 3n + 3
⇒ 14 – 3n = – 150
⇒ 3n= 14 + 150 = 164
∴ n = 1643 = 5423
Here n is not an integer.
∴ -150 is not a term of the given A.P.

Question 7.
Find the 31^st term of an A.P. whose 11^th term is 38 and the 16^th term is 73.
Answer:
Given: An A.P. whose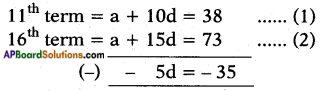

⇒ -5d = -35
⇒ d = −35−5 = 7
Substituting d = 7 in the equation (1)
we get,
a + 10 x 7 = 38
⇒ a + 70 = 38
⇒ a = 38 – 70 = -32
Now, the 31^st term = a + 30d
= (-32) + 30 × 7
= -32 + 210 = 178

Question 8.
If the 3rd and the 9^th terms of an A.P are 4 and -8 respectively, which term of this A.P is zero?
Answer:
Given: An A.P. whose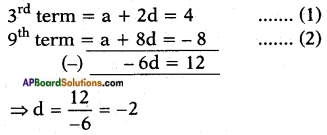

Substituting d = -2 in equation (1) we get
a + 2 × (-2) = 4
⇒ a – 4 = 4
⇒ a = 4 + 4 = 8
Let n^th term of the given A.P be equal to zero.
an = a + (n – 1)d
⇒ 0 = 8 + (n – 1) × (-2)
⇒ 0 = 8 – 2n + 2
⇒ 10 – 2n = 0
⇒ 2n = 10 and n = 102 = 5
∴ The 5^th term of the given A.P is zero.

Question 9.
The 17^th term of an A.P exceeds its 10 term by 7. Find the common difference.
Answer:
Given an A.P in which a₁₇ = a₁₀ + 7
⇒ a₁₇ – a₁₀ = 7
We know that a_n = a + (n – 1)d

⇒ d = 77 = 1

Question 10.
Two APs have the same common difference. The difference between their 100^th terms is 100, what is the difference between their 1000^th terms?
Answer:
Let the first A.P be:
a, a + d, a + 2d, ……..
Second A.P be:
b, b + d, b + 2d, b + 3d, ………
Also, general term, a_n = a + (n – 1)d
Given that, a₁₀₀ – b₁₀₀ = 100
⇒ a + 99d – (b + 99d) = 100
⇒ a – b = 100
Now the difference between their 1000^th terms,

∴ The difference between their 1000^th terms is (a – b) = 100.
Note: If the common difference for any two A.Ps are equal then difference between n^th terms of two A.Ps is same for all natural values of n.

Question 11.
How many three-digit numbers are divisible by 7?
Answer:
The least three digit number is 100.

∴ The least 3 digit number divisible by 7 is 100 + (7 – 2) = 105
The greatest 3 digit number is 999

∴ The greatest 3 digit number divisible by 7 is 999 – 5 = 994.
∴ 3 digit numbers divisible by 7 are
105, 112, 119,….., 994.
a₁ = a = 105; d = 7; a_n = 994
a_n = a + (n – 1)d
⇒ 994 = 105 + (n – 1)7
⇒ (n – 1)7 = 994 – 105
⇒ (n – 1)7 = 889
⇒ n – 1 = 8897 = 127
∴ n = 127 + 1 = 128
∴ There are 128, 3 digit numbers which are divisible by 7.
(or)
 last number – first number 7
999−1007
≃ 128.4 = 128 numbers divisible by 7.

Question 12.
How many multiples of 4 lie between 10 and 250?
Answer:
Given numbers: 10 to 250

∴ Multiples of 4 between 10 and 250 are
First term: 10 + (4 – 2) = 12
Last term: 250 – 2 = 248
∴ 12, 16, 20, 24, ….., 248
a = a₁ = 12; d = 4; a_n = 248
a_n = a + (n – 1)d
248 = 12 + (n – 1) × 4
⇒ (n – 1)4 = 248 – 12
⇒ n – 1 = 2364 = 59
∴ n = 59 + 1 = 60
There are 60 numbers between 10 and 250 which are divisible by 4.

Question 13.
For what value of n, are the n^th terms of two APs: 63, 65, 67, ….. and 3, 10, 17,… equal?
Answer:
Given : The first A.P. is 63, 65, 67, ……
where a = 63, d = a₂ – a₁,
⇒ d = 65 – 63 = 2
and the second A.P. is 3, 10, 17, …….
where a = 3; d = a₂ – a₁ = 10 – 3 = 7
Suppose the nth terms of the two A.Ps are equal, where a_n = a + (n – 1)d
⇒ 63 + (n – 1)2 = 3 + (n – 1)7
⇒ 63 + 2n – 2 = 3 + 7n – 7
⇒ 61 + 2n = 7n – 4
⇒ 7n – 2n = 61 + 4
⇒ 5n = 65
⇒ n = 655 = 13
∴ 13^th terms of the two A.Ps are equal.

Question 14.
Determine the AP whose third term is 16 and the 7^th term exceeds the 5^th term by 12.
Answer:
Given : An A.P in which
a₃ = a + 2d = 16 …… (1)
and a₇ = a₅ + 12
i.e., a + 6d = a + 4d + 12
⇒ 6d – 4d = 12
⇒ 2d = 12
⇒ d = 122 = 6
Substituting d = 6 in equation (1) we get
a + 2 × 6 = 16
⇒ a = 16 – 12 = 4
∴ The series/A.P is
a, a + d, a + 2d, a + 3d, …….
⇒ 4, 4 + 6, 4 + 12, 4 + 18, ……
⇒ A.P.: 4, 10, 16, 22, …….

Question 15.
Find the 20^th term from the end of the AP: 3, 8, 13,…, 253.
Answer:
Given: An A.P: 3, 8, 13, …… , 253
Here a = a₁ = 3
d = a₂ – a₁ = 8 – 3 = 5
a_n = 253, where 253 is the last term
a_n = a + (n – l)d
∴ 253 = 3 + (n – 1)5
⇒ 253 = 3 + 5n – 5
⇒ 5n = 253 + 2
⇒ n = 2555 = 51
∴ The 20^th term from the other end would be
1 + (51 – 20) = 31 + 1 = 32
∴ a₃₂ = 3 + (32 – 1) × 5
= 3 + 31 × 5
= 3 + 155 = 158

Question 16.
The sum of the 4^th and 8^th terms of an AP is 24 and the sum of the 6^th and 10^th terms is 44. Find the first three terms of the AP.
Answer:
Given an A.P in which a₄ + a₈ = 24
⇒ a + 3d + a + 7d = 24
⇒ 2a + 10d = 24
⇒ a + 5d = 12 ……. (1)
and a₆ + a₁₀ = 44
⇒ a + 5d + a + 9d = 44
⇒ 2a + 14d = 44
⇒ a + 7d = 22 ……. (2)
Also a + 5d = 12
⇒ a + 5(5) = 12
⇒ a + 25 = 12
⇒ a = 12 – 25 = -13
∴ The A.P is a, a + d, a + 2d, ……
i.e., – 13, (- 13 + 5), (-13 + 2 × 5)…
⇒ -13, -8, -3, …….

Question 17.
Subba Rao started work in 1995 at an annual salary of Rs. 5000 and received an increment of Rs. 200 each year. In which year did his income reach Rs. 7000?
Answer:
Given: Salary of Subba Rao in 1995 = Rs. 5000
Annual increment = Rs. 200
i.e., His salary increases by Rs. 200 every year.

Clearly 5000, 5200, 5400, forms an A.P in which a = 5000 and d = 200.
Now suppose that his salary reached Rs. 7000 after x – years.
i.e., a_n = 7000
But, a_n = a + (n – 1)d
7000 = 5000 + (n – 1)200
⇒ 7000 – 5000 = (n – 1)200
⇒ n – 1 = 2000200 = 10
⇒ n = 10 + 1
∴ In 11^th year his salary reached Rs. 7000.

AP Board Textbook Solutions PDF for Class 10th Maths

• AP Board Class 10 10th Textbook Solutions PDF
• AP Board Class 10 Maths Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 1 Real Numbers Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 1 Real Numbers Ex 1.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 1 Real Numbers Ex 1.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 1 Real Numbers Ex 1.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 1 Real Numbers Ex 1.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 1 Real Numbers Ex 1.5 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 1 Real Numbers Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 1 Real Numbers InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 2 Sets Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 2 Sets Ex 2.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 2 Sets Ex 2.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 2 Sets Ex 2.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 2 Sets Ex 2.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 2 Sets InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 3 Polynomials Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 3 Polynomials Ex 3.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 3 Polynomials Ex 3.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 3 Polynomials Ex 3.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 3 Polynomials Ex 3.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 3 Polynomials Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 3 Polynomials InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 4 Pair of Linear Equations in Two Variables Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 4 Pair of Linear Equations in Two Variables Ex 4.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 4 Pair of Linear Equations in Two Variables Ex 4.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 4 Pair of Linear Equations in Two Variables Ex 4.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 4 Pair of Linear Equations in Two Variables Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 4 Pair of Linear Equations in Two Variables InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 5 Quadratic Equations Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 5 Quadratic Equations Ex 5.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 5 Quadratic Equations Ex 5.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 5 Quadratic Equations Ex 5.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 5 Quadratic Equations Ex 5.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 5 Quadratic Equations Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 5 Quadratic Equations InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 6 Progressions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 6 Progressions Ex 6.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 6 Progressions Ex 6.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 6 Progressions Ex 6.5 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 6 Progressions Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 6 Progressions InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 7 Coordinate Geometry Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 7 Coordinate Geometry Ex 7.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 7 Coordinate Geometry Ex 7.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 7 Coordinate Geometry Ex 7.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 7 Coordinate Geometry Ex 7.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 7 Coordinate Geometry Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 7 Coordinate Geometry InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 8 Similar Triangles Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 8 Similar Triangles Ex 8.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 8 Similar Triangles Ex 8.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 8 Similar Triangles Ex 8.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 8 Similar Triangles Ex 8.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 8 Similar Triangles Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 8 Similar Triangles InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 9 Tangents and Secants to a Circle Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 9 Tangents and Secants to a Circle Ex 9.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 9 Tangents and Secants to a Circle Ex 9.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 9 Tangents and Secants to a Circle Ex 9.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 9 Tangents and Secants to a Circle Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 9 Tangents and Secants to a Circle InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 10 Mensuration Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 10 Mensuration Ex 10.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 10 Mensuration Ex 10.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 10 Mensuration Ex 10.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 10 Mensuration Ex 10.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 10 Mensuration Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 10 Mensuration InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 11 Trigonometry Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 11 Trigonometry Ex 11.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 11 Trigonometry Ex 11.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 11 Trigonometry Ex 11.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 11 Trigonometry Ex 11.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 11 Trigonometry Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 11 Trigonometry InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 12 Applications of Trigonometry Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 12 Applications of Trigonometry Ex 12.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 12 Applications of Trigonometry Ex 12.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 12 Applications of Trigonometry Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 12 Applications of Trigonometry InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 13 Probability Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 13 Probability Ex 13.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 13 Probability Ex 13.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 13 Probability Optional Exercise Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 13 Probability InText Questions Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 14 Statistics Textbook Solutions PDF

• AP Board Class 10 Maths Chapter 14 Statistics Ex 14.1 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 14 Statistics Ex 14.2 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 14 Statistics Ex 14.3 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 14 Statistics Ex 14.4 Textbook Solutions PDF
• AP Board Class 10 Maths Chapter 14 Statistics InText Questions Textbook Solutions PDF

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks for Exam Preparations

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions can be of great help in your Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 exam preparation. The AP Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks study material, used with the English medium textbooks, can help you complete the entire Class 10th Maths Chapter 6 Progressions Ex 6.2 Books State Board syllabus with maximum efficiency.

FAQs Regarding Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbook Solutions

How to get AP Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbook Answers??

Students can download the Andhra Pradesh Board Class 10 Maths Chapter 6 Progressions Ex 6.2 Answers PDF from the links provided above.

Can we get a Andhra Pradesh State Board Book PDF for all Classes?

Yes you can get Andhra Pradesh Board Text Book PDF for all classes using the links provided in the above article.

Important Terms

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.2, AP Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks, Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.2, Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbook solutions, AP Board Class 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks Solutions, Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.2, AP Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks, Andhra Pradesh State Board STD 10th Maths Chapter 6 Progressions Ex 6.2, Andhra Pradesh State Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Textbook solutions, AP Board STD 10th Maths Chapter 6 Progressions Ex 6.2 Textbooks Solutions,