AP Board Class 10 Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions PDF: Download Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Book Answers ~ HSSlive: Plus One & Plus Two Notes & Solutions for Kerala State Board

Thursday, June 9, 2022

AP Board Class 10 Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions PDF: Download Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Book Answers

June 09, 2022 AP State Board No comments

AP Board Class 10 Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions PDF: Download Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Book Answers

AP Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks Solutions and answers for students are now available in pdf format. Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Book answers and solutions are one of the most important study materials for any student. The Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.3 books are published by the Andhra Pradesh Board Publishers. These Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 textbooks are prepared by a group of expert faculty members. Students can download these AP Board STD 10th Maths Chapter 6 Progressions Ex 6.3 book solutions pdf online from this page.

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks Solutions PDF

Andhra Pradesh State Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Books Solutions with Answers are prepared and published by the Andhra Pradesh Board Publishers. It is an autonomous organization to advise and assist qualitative improvements in school education. If you are in search of AP Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Books Answers Solutions, then you are in the right place. Here is a complete hub of Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.3 solutions that are available here for free PDF downloads to help students for their adequate preparation. You can find all the subjects of Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks. These Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks Solutions English PDF will be helpful for effective education, and a maximum number of questions in exams are chosen from Andhra Pradesh Board.

Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Books Solutions

Board	AP Board
Materials	Textbook Solutions/Guide
Format	DOC/PDF
Class	10th
Subject	Maths
Chapters	Maths Chapter 6 Progressions Ex 6.3
Provider	Hsslive

How to download Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions Answers PDF Online?

Visit our website - Hsslive
Click on the Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Answers.
Look for your Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks PDF.
Now download or read the Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions for PDF Free.

AP Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks Solutions with Answer PDF Download

Find below the list of all AP Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions for PDF’s for you to download and prepare for the upcoming exams:

10th Class Maths 6th Lesson Progressions Ex 6.3 Textbook Questions and Answers

Question 1.
Find the sum of the following APs:
i) 2, 7, 12,…, to 10 terms.
Answer:
Given A.P: 2, 7, 12, …… to 10 terms
a = 2; d = a₂ – a₁ = 7 – 2 = 5; n = 10
S_n = 𝑛2[2a + (n – 1)d]
∴ S₁₀ = 102[2 × 2 + (10 – 1)5]
= 5 [4 + 9 × 5]
= 5 [4 + 45]
= 5 × 49
= 245

ii) -37, -33, -29,…, to 12 terms.
Answer:
Given A.P: -37, -33, -29,…, to 12 terms.
a = -37; d = a₂ – a₁ = (-33) – (-37) = -33 + 37 = 4; n = 12
S_n = 𝑛2[2a + (n – 1)d]
∴ S₁₂ = 122[2 × (-37) + (12 – 1)4]
= 6 [-74 + 11 × 4]
= 6 [-74 + 44]
= 6 × (-30)
= -180

iii) 0.6, 1.7, 2.8,…, to 100 terms.
Answer:
Given A.P : 0.6, 1.7, 2.8,…. S₁₀₀
a = 0.6; d = a₂ – a₁ = 1.7 – 0.6 = 1.1; n = 100
S_n = 𝑛2[2a + (n – 1)d]
∴ S₁₀₀ = 1002[2 × 0.6 + (100 – 1)1.1]
= 50 [1.2 + 99 × 1.1]
= 50 [1.2 + 108.9]
= 50 × 110.1
= 5505

iv) 115, 112, 110,…, to 11 terms.
Answer:
Given A.P: 115, 112, 110,…, S₁₁
a = 115; d = a₂ – a₁ = 112 – 115 = 5−460 = 160; n = 11
S_n = 𝑛2[2a + (n – 1)d]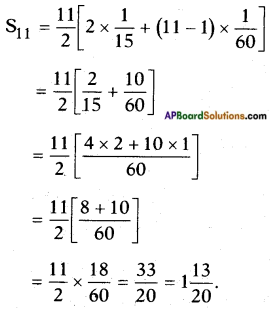

2. Find the sums given below =:
i) 7 + 1012 + 14 + …. + 84
Answer:
Given A.P : 7 + 1012 + 14 + …. + 84
a = 7; d = a₂ – a₁ = 1012 – 7 = 312 and the last term l = a_n = 84
But, a_n = a + (n – 1) d
∴ 84 = 7 + (n – 1) 312
⇒ 84 – 7 = (n – 1) × 72
⇒ n – 1 = 77 × 27 = 22
⇒ n = 22 + 1 = 23
Now, S_n = 𝑛2(a + l) where a = 7; l = 84
S₂₃ = 232(7 + 84)
= 232 × 91
= 20932
= 104612

ii) 34 + 32 + 30 + … + 10
Answer:
Given A.P: 34 + 32 + 30 + … + 10
a = 34; d = a₂ – a₁ = 32 – 34 = -2 and the last term l = a_n = 10
But, a_n = a + (n – 1) d
∴ 10 = 34 + (n – 1) (-2)
⇒ 10 – 34 = -2n + 2
⇒ -2n = -24 – 2
⇒ n = −26−2 = 13
∴ n = 13
Also, S_n = 𝑛2(a + l)
where a = 34; l = 10
S₁₃ = 132(34 + 10)
= 132 × 44
= 13 × 22
= 286

iii) -5 + (-8) + (-11) + … + (-230)
Answer:
Given A.P: -5 + (-8) + (-11) + … + (-230)
Here first term, a = -5;
d = a₂ – a₁ = (-8) – (-5) = -8 + 5 = -3 and the last term l = a_n = 10
But, a_n = a + (n – 1) d
∴ (-230) = -5 + (n – 1) (-3)
⇒ -230 + 5 = -3n + 3
⇒ -3n + 3 = -225
⇒ -3n = -225 – 3
⇒ 3n = 228
⇒ n = 2283 = 76
∴ n = 76
Now, S_n = 𝑛2(a + l)
where a = -5; l = -230
S₇₆ = 762((-5) + (-230))
= 38 × (-235)
= -8930

Question 3.
In an AP:
i) Given a = 5, d = 3, a_n = 50. find n and S_n.
Answer:
Given :
a = 5; d = 3;
a_n = a + (n – 1)d = 50
⇒ 50 = 5 + (n – 1) 3
⇒ 50 – 5 = 3n – 3
⇒ 3n = 45 + 3
⇒ n = 483 = 16
Now, S_n = 𝑛2(a + l)
S₁₆ = 162(5 + 50)
= 38 × 55
= 440

ii) Given a = 7, a₁₃ = 35, find d and S₁₃.
Answer:
Given: a = 7;
a₁₃ = a + 12d = 35
⇒ 7 + 12d = 35
⇒ 12d = 35 – 7
⇒ n = 2812 = 73
Now, S_n = 𝑛2(a + l)
S₁₃ = 132(7 + 35)
= 132 × 42
= 13 × 21
= 273

iii) Given a₁₂ = 37, d = 3 find a and S₁₂.
Answer:
Given:
a₁₂ = a + 11d = 37
d = 3
So, a₁₂ = a + 11 × 3 = 37
⇒ a + 33 = 37
⇒ a = 37 – 33 = 4
Now, S_n = 𝑛2(a + l)
S₁₂ = 122(4 + 37)
= 6 × 41
= 246

iv) Given a₃ = 15, S₁₀ = 125, find d and a₁₀.
Answer:
Given:
a₃ = a + 2d = 15
⇒ a = 15 – 2d ……… (1)
S₁₀ = 125 but take S₁₀ as 175
i.e., S₁₀ = 175
We know that,

⇒ 35 = 2 (15 – 2d) + 9d [∵ a = 15 – 2d]
⇒ 35 = 30 – 4d + 9d
⇒ 35 – 30 = 5d
⇒ d = 55 = 1
Substituting d = 1 in equation (1) we get
a = 15 – 2 × 1 = 15 – 2 = 13
Now, a_n = a + (n – 1) d
a₁₀ = a + 9d = 13 + 9 × 1 = 13 + 9 = 22
∴ a₁₀ = 22; d = 1

v) Given a = 2, d = 8, S_n = 90, find n and a_n.
Answer:
Given a = 2, d = 8, S_n = 90
S_n = 𝑛2[2a + (n – 1)d]

⇒ 90 = 2n [2n – 1]
⇒ 4n² – 2n = 90
⇒ 4n² – 2n – 90 = 0
⇒ 2(2n²– n – 45) = 0
⇒ 2n² – n – 45 = 0
⇒ 2n² -10n + 9n – 45 = 0
⇒ 2n(n – 5) + 9(n – 5) = 0
⇒ (n – 5)(2n + 9) = 0
⇒ n – 5 = 0 (or) 2n + 9 = 0
⇒ n = 5 (or) n = −92 (discarded)
∴ n = 5
Now a_n = a₅ = a + 4d = 2 + 4 x 8
= 2 + 32 = 34

vi) Given a_n = 4, d = 2, S_n = -14, find n and a.
Answer:
Given a_n = a + (n – 1) d = 4 ……. (1)
d = 2; S_n = – 14
From (1); a + (n – 1) 2 = 4
a = 4 – 2n + 2
a = 6 – 2n
Given a = 2, d = 8, S_n = 90
S_n = 𝑛2[a + a_n]
-14 = 𝑛2[(6-2n) + 4] [∵ a = 6 – 2n]
-14 × 2 = n (10 – 2n)
⇒ 10n – 2n² = – 28
⇒ 2n² – 10n – 28 = 0
⇒ n² – 5n – 14 = 0
⇒ n² – 7n + 2n – 14 = 0
⇒ n (n – 7) + 2 (n – 7) = 0
⇒ (n – 7) (n + 2) = 0
⇒ n = 7 (or) n = – 2
∴ n = 7
Now a = 6 – 2n = 6 – 2 × 7
= 6 – 14 = -8
∴ a = – 8; n = 7

vii) Given l = 28, S = 144, and there are total 9 terms. Find a.
Answer:
Given:
l = a₉ = a + 8d = 28 and S₉ = 144 But,
Now, S_n = 𝑛2(a + l)
144 = 92(a + 28)
⇒ 144 × 29 = a + 28
⇒ a + 28 = 32
⇒ a = 4

Question 4.
The first and the last terms of an A.P are 17 and 350 respectively. If the common difference is 9, how many terms are there and what is their sum?
Answer:
Given A.P in which a = 17
Last term = l = 350
Common difference, d = 9
We know that, a_n = a + (n – 1) d
350 = 17 + (n- 1) 9
⇒ 350 = 17 + 9n – 9
⇒ 9n = 350 – 8
⇒ n = 3429 = 38
Now, S_n = 𝑛2(a + l)
S₃₈ = 382(17 + 350)
= 19 × 367 = 6973
∴ n = 38; S_n = 6973

Question 5.
Find the sum of first 51 terms of an AP whose second and third terms are 14 and 18 respectively.
Answer:
Given A.P in which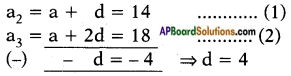

Substituting d = 4 in equation (1),
we get a + 4 = 14
⇒ a = 14 – 4 = 10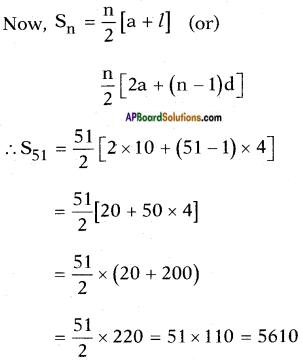

Question 6.
If the sum of first 7 terms of an AP is 49 and that of 17 terms is 289, find the sum of first n terms.
Answer:
Given :
A.P such that S₇ = 49; S₁₇ = 289
We know that,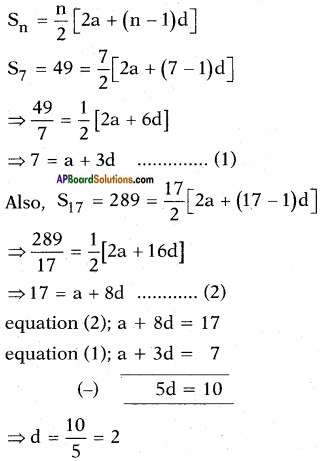

Substituting d = 2 in equation (1), we get,
a + 3 × 2 = 7
⇒ a = 7 – 6 = 1
∴ a = 1; d = 2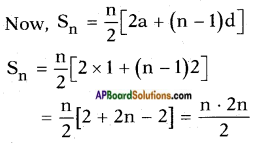

∴ Sum of first n terms S_n = n².
Shortcut: S₇ = 49 = 7²
S₁₇ = 289 = 17²
∴ S_n = n²

Question 7.
Show that a₁, a₂ …,a_n, …. form an AP where a_n is defined as below:
i) a = 3 + 4n
ii) a_n = 9 – 5n. Also find the sum of the first 15 terms in each case.
Answer:
Given a_n = 3 + 4n
Then a₁ = 3 + 4 × l = 3 + 4 = 7
a₂ = 3 + 4 × 2 = 3 + 8 = 11
a₃ = 3 + 4 × 3 = 3 + 12 = 15
a₄ = 3 + 4 × 4 = 3 + 16 = 19
Now the pattern is 7, 11, 15, ……
where a = a₁ = 7; a₂ = 11; a₃ = 15, ….. and
a₂ – a₁ = 11 – 7 = 4;
a₃ – a₂ = 15 – 11 = 4;
Here d = 4
Hence a₁, a₂, ….., a_n ….. forms an A.P.

ii) a_n = 9 – 5n
Given: a_n = 9 – 5n.
a₁ = 9 – 5 × l = 9 – 5 = 4
a₂ = 9 – 5 × 2 = 9 – 10 = -1
a₃ = 9 – 5 × 3 = 9 – 15 = -6
a₄ = 9 – 5 × 4 = 9 – 20 = -11
Also
a₂ – a₁ = -1 – 4 = -5;
a₃ – a₂ = -6 – (-1) = – 6 + 1 = -5
a₄ – a₃ = -11 – (-6) = -11 + 6 = -5
∴ d = a₂ – a₁ = a₃ – a₂ = a₄ – a₃ = …. = -5
Thus the difference between any two successive terms is constant (or) starting from the second term, each term is obtained by adding a fixed number ‘-5’ to its preceding term.
Hence {a_n} forms an A.P.

Question 8.
If the sum of the first n terms of an AP is 4n – n², what is the first term (remember the first term is S₁)? What is the sum of first two terms? What is the second term? Similarly, find the 3^rd, the 10^th and the n^th terms.
Answer:
Given an A.P in which S_n = 4n – n²
Taking n = 1 we get
S₁ = 4 × 1- 12 = 4 – 1 = 3
n = 2; S₂ = a₁ + a₂ = 4 × 2 – 2² = 8 – 4 = 4
n = 3; S₃ = a₁ + a₂ + a₃ = 4 × 3 -3² = 12 – 9 = 3
n = 4; S₄ = a₁ + a₂ + a₃ + a₄ = 4 × 4 – 4² = 16 – 16 = 0
Hence, S₁ = a₁ = 3
a₂ = S₂ – S₁ = 4 – 3 = 1
a₃ = S₃ – S₂ = 3 – 4 = -1
a₄ = S₄ – S₃ = 0 – 3 = -3
So, d = a₂ – a₁ = l – 3 = -2
Now, a₁₀ = a + 9d [∵ a_n = a + (n – 1) d]
= 3 + 9 × (- 2)
= 3 – 18 = -15
a_n = 3 + (n – 1) × (-2)
= 3 – 2n + 2
= 5 – 2n

Question 9.
Find the sum of the first 40 positive integers divisible by 6.
Answer:
The given numbers are the first 40 positive multiples of 6
⇒ 6 × 1, 6 × 2, 6 × 3, ….., 6 × 40
⇒ 6, 12, 18, ….. 240
S_n = 𝑛2(a + l)
S₄₀ = 402(6 + 240)
= 20 × 246
= 4920
∴ S₄₀ = 4920

Question 10.
A sum of Rs. 700 is to be used to give seven cash prizes to students of a school for their overall academic performance. If each prize is Rs. 20 less than its preceding prize, find the value of each of the prizes.
Answer:
Given:
Total/Sum of all cash prizes = Rs. 700
Each prize differs by Rs. 20
Let the prizes (in ascending order) be x, x + 20, x + 40, x + 60, x + 80, x + 100, x + 120
∴ Sum of the prizes = S₇ = 𝑛2(a + l)
⇒ 700 = 72[x + x + 120]
⇒ 700 × 27 = 2x + 120
⇒ 100 = x + 60
⇒ x = 100 – 60 = 40
∴ The prizes are 160, 140, 120, 100, 80, 60, 40.

Question 11.
In a school, students thought of plant¬ing trees in and around the school to reduce air pollution. It was decided that the number of trees, that each section of each class will plant, will be the same as the class, in which they are studying, e.g., a section of Class I will plant 1 tree, a section of Class II will plant 2 trees and so on till Class XII. There are three sections of each class. How many trees will be planted by the students?
Answer:
Given: Classes: From I to XII
Section: 3 in each class.
∴ Trees planted by each class=3 × class number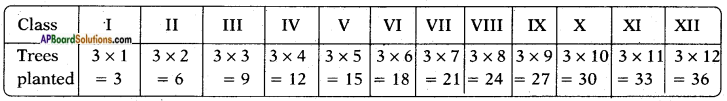

∴ Total trees planted = 3 + 6 + 9 + 12 + …… + 36 is an A.P.
Here, a = 3 and l = 36; n = 12
∴ S_n = 𝑛2(a + l)
S₁₂ = 122[3 + 36]
= 6 × 39
= 234
∴ Total plants = 234

Question 12.
A spiral is made up of successive semicircles, with centres alternately at A and B, starting with centre at A, of radii 0.5 cm, 1.0 cm, 1.5 cm, 2.0 cm, … as shown in figure. What is the total length of such a spiral made up of thirteen
consecutive semicircles? (Take π = 227)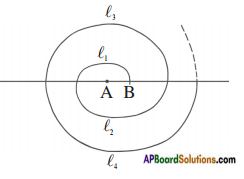

[Hint: Length of successive semicircles is l₁, l₂, l₃, l₄,….. with centres at A, B, A, B,…, respectively.]
Answer:
Given: l₁, l₂, l₃, l₄,….., l₁₃ are the semicircles with centres alternately at A and B; with radii
r₁ = 0.5 cm [1 × 0.5]
r₂ = 1.0 cm [2 × 0.5]
r₃ = 1.5 cm [3 × 0.5]
r₄ = 2.0 cm [4 × 0.5] [∵ Radii are in A.P. as aj = 0.5 and d = 0.5]
……………………………
r₁₃ = 13 × 0.5 = 6.5
Now, the total length of the spiral = l₁ + l₂ + l₃ + l₄ + ….. + l₁₃ [∵ 13 given]
But circumference of a semi-cirle is πr.
∴ Total length of the spiral = π × 0.5 + π × 1.0 + ………. + π × 6.5
= π × 12[l + 2 + 3 + ….. + 13]
[∵ Sum of the first n – natural numbers is 𝑛(𝑛+1)2
= 227×12×13×142
= 11 × 13
= 143 cm.

Question 13.
200 logs are stacked in the following manner: 20 logs in the bottom row, 19 in the next row, 18 in the row next to it and so on. In how many rows are the 200 logs placed and how many logs are in the top row?

Answer:
Given: Total logs = 200
Number of logs stacked in the first row = 20
Number of logs stacked in the second row = 19
Number of logs stacked in the third row = 18
The number series is 20, 19, 18,….. is an A.P where a = 20 and
d = a₂ – a₁ = 19 – 20 = -1
Also, S_n = 200

400 = 41n – n²
⇒ n² – 41n + 400 = 0
⇒ n² – 25n – 16n + 400 = 0
⇒ n(n – 25) – 16(n – 25) = 0
⇒ (n – 25) (n – 16) = 0
⇒ n – 25 (or) 16
There can’t be 25 rows as we are starting with 20 logs in the first row.
∴ Number of rows must be 16.
∴ n = 16

Question 14.
In a bucket and ball race, a bucket is placed at the starting point, which is 5 m from the first ball, and the other balls are placed 3 m apart in a straight line. There are ten balls in the line.

A competitor starts from the bucket, picks up the nearest ball, runs back with it, drops it in the bucket, runs back to pick up the next ball, runs to the bucket to drop it in, and she continues in the same way until all the balls are in the bucket. What is the total distance the competitor has to run?
[Hint: To pick up the first ball and the second ball, the total distance (in metres) run by a competitor is 2 × 5 + 2 × (5 + 3)]
Answer:
Given: Balls are placed at an equal distance of 3 m from one another.
Distance of first ball from the bucket = 5 m
Distance of second ball from the bucket = 5 + 3 = 8 m (5 + 1 × 3)
Distance of third ball from the bucket = 8 + 3 = 11 m (5 + 2 × 3)
Distance of fourth ball from the bucket = 11 + 3 = 14 m (5 + 3 × 3)
………………………………
∴ Distance of the tenth ball from the bucket = 5 + 9 × 3 = 5 + 27 = 32 m.
1st ball: Distance covered by the competitor in picking up and dropping it in the bucket = 2 × 5 = 10 m.
2nd ball: Distance covered by the competitor in picking up and dropping it in the bucket = 2 × 8 = 16 m.
3rd ball: Distance covered by the competitor in picking up and dropping it in the bucket = 2 × 11 = 22 m.
………………………………
10th ball: Distance covered by the competitor in picking up and dropping it in the bucket = 2 × 32 = 64 m.
Total distance = 10 m + 16 m + 22 m + …… + 64 m.
Clearly, this is an A.P in which a = 10; d = a₂ – a₁ = 16 – 10 = 6 and n = 10.
∴ S_n = 𝑛2[2a + (n – 1)d]
∴ S₁₀ = 102[2 × 10 + (10 – 1)6]
= 5 [20 + 54]
= 5 × 74
= 370 m
∴ Total distance = 370 m.

AP Board Textbook Solutions PDF for Class 10th Maths

AP Board Class 10 Maths Chapter 1 Real Numbers Textbook Solutions PDF

AP Board Class 10 Maths Chapter 2 Sets Textbook Solutions PDF

AP Board Class 10 Maths Chapter 3 Polynomials Textbook Solutions PDF

AP Board Class 10 Maths Chapter 4 Pair of Linear Equations in Two Variables Textbook Solutions PDF

AP Board Class 10 Maths Chapter 5 Quadratic Equations Textbook Solutions PDF

AP Board Class 10 Maths Chapter 6 Progressions Textbook Solutions PDF

AP Board Class 10 Maths Chapter 7 Coordinate Geometry Textbook Solutions PDF

AP Board Class 10 Maths Chapter 8 Similar Triangles Textbook Solutions PDF

AP Board Class 10 Maths Chapter 9 Tangents and Secants to a Circle Textbook Solutions PDF

AP Board Class 10 Maths Chapter 10 Mensuration Textbook Solutions PDF

AP Board Class 10 Maths Chapter 11 Trigonometry Textbook Solutions PDF

AP Board Class 10 Maths Chapter 12 Applications of Trigonometry Textbook Solutions PDF

AP Board Class 10 Maths Chapter 13 Probability Textbook Solutions PDF

AP Board Class 10 Maths Chapter 14 Statistics Textbook Solutions PDF

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks for Exam Preparations

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions can be of great help in your Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 exam preparation. The AP Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks study material, used with the English medium textbooks, can help you complete the entire Class 10th Maths Chapter 6 Progressions Ex 6.3 Books State Board syllabus with maximum efficiency.

FAQs Regarding Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbook Solutions

How to get AP Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbook Answers??

Students can download the Andhra Pradesh Board Class 10 Maths Chapter 6 Progressions Ex 6.3 Answers PDF from the links provided above.

Can we get a Andhra Pradesh State Board Book PDF for all Classes?

Yes you can get Andhra Pradesh Board Text Book PDF for all classes using the links provided in the above article.

Important Terms

Andhra Pradesh Board Class 10th Maths Chapter 6 Progressions Ex 6.3, AP Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks, Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.3, Andhra Pradesh State Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbook solutions, AP Board Class 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks Solutions, Andhra Pradesh Board STD 10th Maths Chapter 6 Progressions Ex 6.3, AP Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks, Andhra Pradesh State Board STD 10th Maths Chapter 6 Progressions Ex 6.3, Andhra Pradesh State Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Textbook solutions, AP Board STD 10th Maths Chapter 6 Progressions Ex 6.3 Textbooks Solutions,

HSSlive: Plus One & Plus Two Notes & Solutions for Kerala State Board

Hsslive.co.in: Kerala Higher Secondary News, Plus Two Notes, Plus One Notes, Plus two study material, Higher Secondary Question Paper.

Thursday, June 9, 2022